Approximate Analysis of Indeterminate Structures

1. Conventional design process is normally based on the ‘local’ structural elements (column, beam, floor slabs, wall, etc.)
2. But theoretical and experimental studies have shown that structural systems cannot be considered to be a simple collection of individual elements
3. The responses of the structure is often more than the ‘sum’ of the responses of individual elements since structural integrity ensures that the elements work together, producing global responses through the complex interaction of its elements
4. Hence ‘local’ and ‘global’ approaches are necessary for proper design

5. Global analysis carried out on two levels:
   1. A ‘numerically exact’ analysis using finite element method or another mathematical procedure, in which each of the element of the system is described by a mathematical equation and joined together at various points (or along edges) by proper boundary or continuity conditions (MATHEMATICA) - This procedure is quit complex and may have in-built data errors
   2. The second procedure is a simplified or approximated procedure, which reduces the complex structural system to a much simpler system that could be handled easily by simple calculations; this will be the subject of our study in this set of lectures
6. Approximate global structural analysis of a complex structure will contain:
   1. Reduction of the complex system into an equivalent simple system to carry out
      1. Stability analysis
      2. Frequency analysis
      3. Elementary structural analysis - this will form the basis of our study in these lectures
7. A model - which is a determinate structure - must be developed for analysis
8. Results obtained from this approximated model compares favorably with the correct results
9. This study makes the transition from determinate to indeterminate structural analysis
10. Preliminary design of all structures is based on this approximate analysis
11. Structures considered include: Indeterminate Trusses, Portals and Trussed Frames, Multi-story frames
12. Loads considered include: Vertical and horizontal loads

Vertical Loads on Building Frames

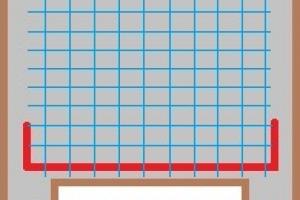
• Building frames often consist of girders that are rigidly connected to columns so that the entire structure is better able to resist the effects of lateral forces generated due to wind and earthquake forces. Most of the simplifying assumptions made to reduce a frame from an indeterminate structure to one that is statically determinate depends on the way that the structure deforms under the given loads

Assumptions:

1. There is zero moment in the horizontal beam girder at a distance of 0.1L from the left and right supports; and
2. The girder does not support any axial force

PORTALS AND TRUSSED FRAME STRUCTURES - VERTICAL AND LATERAL LOADS

• When a portal is used to span large distances, a truss may be used in place of the top horizontal girder - Such structures are used for large bridges, large auditoriums and industrial structures such as mill bents, ware houses, and others (as transverse frames)
• In all cases, the suspended truss is assumed to be pin connected at its point of attachment to the columns
• Furthermore, the truss is assumed to keep the columns straight within the region of attachment, when the portal is subjected to a side-sway.
• For pin-supported columns, assume that the horizontal reactions are equal

For fully fixed-supported columns, assume that the horizontal reactions are equal and an inflection point occurs on each column, midway between the base of the column and the lowest point of truss member connection to the frame - For partially fixed-supported columns (at bottom), the inflection points occur on columns at one-third height from the base

LATERAL LOADS ON BUILDING FRAMES

• Method I - Portal Frame Method: Inflection points are assumed to occur at the middle points of beams and columns (earlier assumptions made for partial fixity at base are also valid) - At any given floor level, interior columns are assumed to carry twice the horizontal shear carried by the exterior columns.

• Method II - Cantilever frame method: Hinges are placed at the center of each girder and column (earlier assumptions made for partial fixity at base are also valid) - The axial stress in a column is proportional to its distance from the centroid of the cross-sectional areas of the columns at a given floor level; since stress equals force per area, in the case of columns having equal cross-sectional areas, the force in a column is also proportional to its distance from the centroid of the column areas.